1. Merge K Sorted Linked Lists
You are given an array of k linked-lists lists, each linked-list is sorted in ascending order.
Merge all the linked-lists into one sorted linked-list and return it.
Example 1:
Input: lists = [[1,4,5],[1,3,4],[2,6]] Output: [1,1,2,3,4,4,5,6] Explanation: The linked-lists are: [ 1->4->5, 1->3->4, 2->6 ] merging them into one sorted list: 1->1->2->3->4->4->5->6
Example 2:
Input: lists = [] Output: []
Example 3:
Input: lists = [[]] Output: []
Constraints:
- k == lists.length
- 0 <= k <= 104
- 0 <= lists[i].length <= 500
- -104 <= lists[i][j] <= 104
- lists[i] is sorted in ascending order.
- The sum of lists[i].length will not exceed 104.
2. Course Schedule II
There are a total of numCourses courses you have to take, labeled from 0 to numCourses - 1. You are given an array prerequisites where prerequisites[i] = [ai, bi] indicates that you must take course bi first if you want to take course ai.
- For example, the pair [0, 1], indicates that to take course 0 you have to first take course 1.
Return the ordering of courses you should take to finish all courses. If there are many valid answers, return any of them. If it is impossible to finish all courses, return an empty array.
Example 1:
Input: numCourses = 2, prerequisites = [[1,0]] Output: [0,1] Explanation: There are a total of 2 courses to take. To take course 1 you should have finished course 0. So the correct course order is [0,1].
Example 2:
Input: numCourses = 4, prerequisites = [[1,0],[2,0],[3,1],[3,2]] Output: [0,2,1,3] Explanation: There are a total of 4 courses to take. To take course 3 you should have finished both courses 1 and 2. Both courses 1 and 2 should be taken after you finished course 0. So one correct course order is [0,1,2,3]. Another correct ordering is [0,2,1,3].
Example 3:
Input: numCourses = 1, prerequisites = [] Output: [0]
Constraints:
- 1 <= numCourses <= 2000
- 0 <= prerequisites.length <= numCourses * (numCourses - 1)
- prerequisites[i].length == 2
- 0 <= ai, bi < numCourses
- ai != bi
- All the pairs [ai, bi] are distinct.
3. Course Schedule Feasibility Check
There are a total of numCourses courses you have to take, labeled from 0 to numCourses - 1. You are given an array prerequisites where prerequisites[i] = [ai, bi] indicates that you must take course bi first if you want to take course ai.
- For example, the pair [0, 1], indicates that to take course 0 you have to first take course 1.
Return true if you can finish all courses. Otherwise, return false.
Example 1:
Input: numCourses = 2, prerequisites = [[1,0]] Output: true Explanation: There are a total of 2 courses to take. To take course 1 you should have finished course 0. So it is possible.
Example 2:
Input: numCourses = 2, prerequisites = [[1,0],[0,1]] Output: false Explanation: There are a total of 2 courses to take. To take course 1 you should have finished course 0, and to take course 0 you should also have finished course 1. So it is impossible.
Constraints:
- 1 <= numCourses <= 2000
- 0 <= prerequisites.length <= 5000
- prerequisites[i].length == 2
- 0 <= ai, bi < numCourses
- All the pairs prerequisites[i] are unique.
4. Shortest Path in a Grid with Obstacles Elimination
You are given an m x n integer matrix grid where each cell is either 0 (empty) or 1 (obstacle). You can move up, down, left, or right from and to an empty cell in one step.
Return the minimum number of steps to walk from the upper left corner (0, 0) to the lower right corner (m - 1, n - 1) given that you can eliminate at most k obstacles. If it is not possible to find such walk return -1.
Example 1:

Input: grid = [[0,0,0],[1,1,0],[0,0,0],[0,1,1],[0,0,0]], k = 1 Output: 6 Explanation: The shortest path without eliminating any obstacle is 10. The shortest path with one obstacle elimination at position (3,2) is 6. Such path is (0,0) -> (0,1) -> (0,2) -> (1,2) -> (2,2) -> (3,2) -> (4,2).
Example 2:

Input: grid = [[0,1,1],[1,1,1],[1,0,0]], k = 1 Output: -1 Explanation: We need to eliminate at least two obstacles to find such a walk.
Constraints:
- m == grid.length
- n == grid[i].length
- 1 <= m, n <= 40
- 1 <= k <= m * n
- grid[i][j] is either 0 or 1.
- grid[0][0] == grid[m - 1][n - 1] == 0
5. Word Search II on a Board
Given an m x n board of characters and a list of strings words, return all words on the board.
Each word must be constructed from letters of sequentially adjacent cells, where adjacent cells are horizontally or vertically neighboring. The same letter cell may not be used more than once in a word.
Example 1:
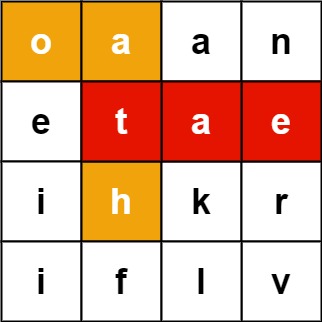
Input: board = [["o","a","a","n"],["e","t","a","e"],["i","h","k","r"],["i","f","l","v"]], words = ["oath","pea","eat","rain"] Output: ["eat","oath"]
Example 2:
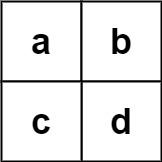
Input: board = [["a","b"],["c","d"]], words = ["abcb"] Output: []
Constraints:
- m == board.length
- n == board[i].length
- 1 <= m, n <= 12
- board[i][j] is a lowercase English letter.
- 1 <= words.length <= 3 * 104
- 1 <= words[i].length <= 10
- words[i] consists of lowercase English letters.
- All the strings of words are unique.


